For a precise definition: homogeneity means the universe has translational invariance and isotropy means it has rotational invariance.
In more lay terms: homogeneous means you can move from one random point in the universe to another and everything looks exactly the same. Look at the sky at night with all the stars and galaxies that dot the sky. If you moved to any other random place in the universe, the odds are it would look very similar. This experimentally verified fact means the universe is nearly homogeneous.
In lay terms, isotropy means you could turn any direction and the universe looks exactly the same. Look at the sky in the Northern, then Southern Hemisphere and statistically they look nearly identical. Look in the USA, look in China, etc...
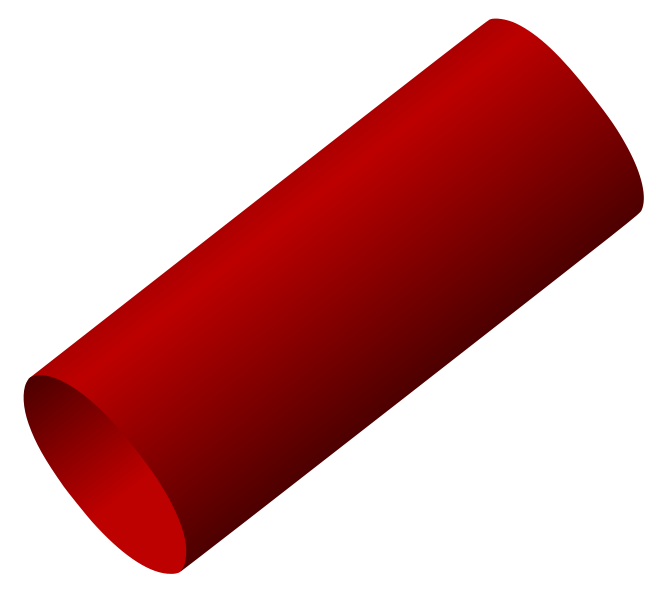
The cylinder above is another example of being homogeneous. If the cylinder was infinity long, every point would look like any other. However the cylinder is not isotropic. If you look down one direction you would see space extends to infinity. In the other direction space extends only 2*pi*R. The cylinder is homogeneous and not isotropic whereas are universe is nearly both.
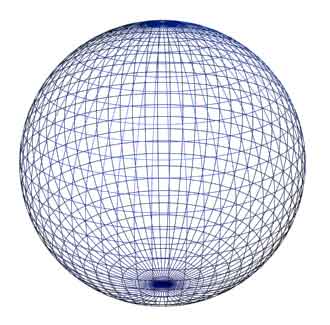
The surface of a sphere, however, is perfectly homogeneous and isotropic so it makes sense when deriving the geometry of the universe to start with that then generalize in a way that matches relativity. This is often what is done.
Any questions?
Hello,
ReplyDeleteI am going to relate about fractality on my blog, in the context of a new system.
Cordially
Cartesian
Cartesian, awesome, I will make sure to follow along.
ReplyDeleteI'm not sure I've gotten it, but here goes a question.
ReplyDeleteIf we take the cylinder and connect the top and bottom ends, we've created a torus (doughnut). Does the torus satisfy the requirements of homogeneity and isotropicity? (new word?).
From the surface of the torus, every point would look like every other point (homogeneity), and if you look in different directions, everything would look the same (isotropic). Is this right?
The launch of the Wilkinson Microwave Anisotropy Probe in 2001 to study the Cosmic Microwave Background (radiation from the big band) has produced data suggesting that if we venture far enough into space, we may return to our starting location.
The plausability of a torus-shaped universe led Stephen Hawking to say to Homer, "Your theory of a doughnut shaped universe is intriguing. I may have to steal it."
Dave, I'm glad you are thinking of these shapes and you are on the right track.
ReplyDeleteIt is true theorists appeal to tori every so often in describing our universe since they have such interesting properties and the hope is the "topology" of the torus of the torus will eventually make some interesting prediction other topologies do not predict. So far this is all speculative, but there may be something there.
Furthermore, I believe the torus a favorite shape to use to curl up extra dimensions in string theory.
Unfortunately a torus is not quite completely homogeneous, but in many ways, as you suggested, is more homogeneous than a cylinder.
This is hard to show without appealing to a picture, but if you are living on the inside edge of the torus you experience negative curvature and if you live on the outside edge you experience positive curvature. Another way to say this is on the inside it looks like you live in a half pipe and on the outside it looks like you live on the top of a hill with all sides pointing downward.
Using a sphere in standard cosmology is what is done because was have every reason to believe the universe is homogeneous and isotropic at every point.
However, going back to the theorists and the torus, if the torus was big enough, really really big enough, it would appear homogeneous and isotropic to creatures living on it because they can't see far enough away to see points that look different than theirs.
Hence, this is an option explored in theory. What if it only looks homogeneous and isotropic around us because the torus is so big? But again, they being good scientists need to work out the details of how to test such a hypothesis. They are trying to do that, but so far the best model is still a generalized sphere.
Maybe one day we will find out it is like a really, really big torus however. It's possible and to many theorists, like Hawking a very interesting idea.
Joseph,
ReplyDeleteGot it. A torus lacks homogeneity because the curvature of the inside and outside surfaces would be different.
Dave C, yes that is correct.
ReplyDeleteexplanation with these two diagrams is really great, and thanks for explanation
ReplyDeleteHi so what does isotropic cylinder will look like? Thank you!
ReplyDeleteI believe there cannot be an isotropic cylinder. The only examples of isotropic and respectively homogeneous surfaces I can think of are a sphere and a plane.
ReplyDelete